
You can apply the methods used to solve square and cube root equations to solving
equations with roots of any index. To undo an
n
th root, raise to the
n
th power.
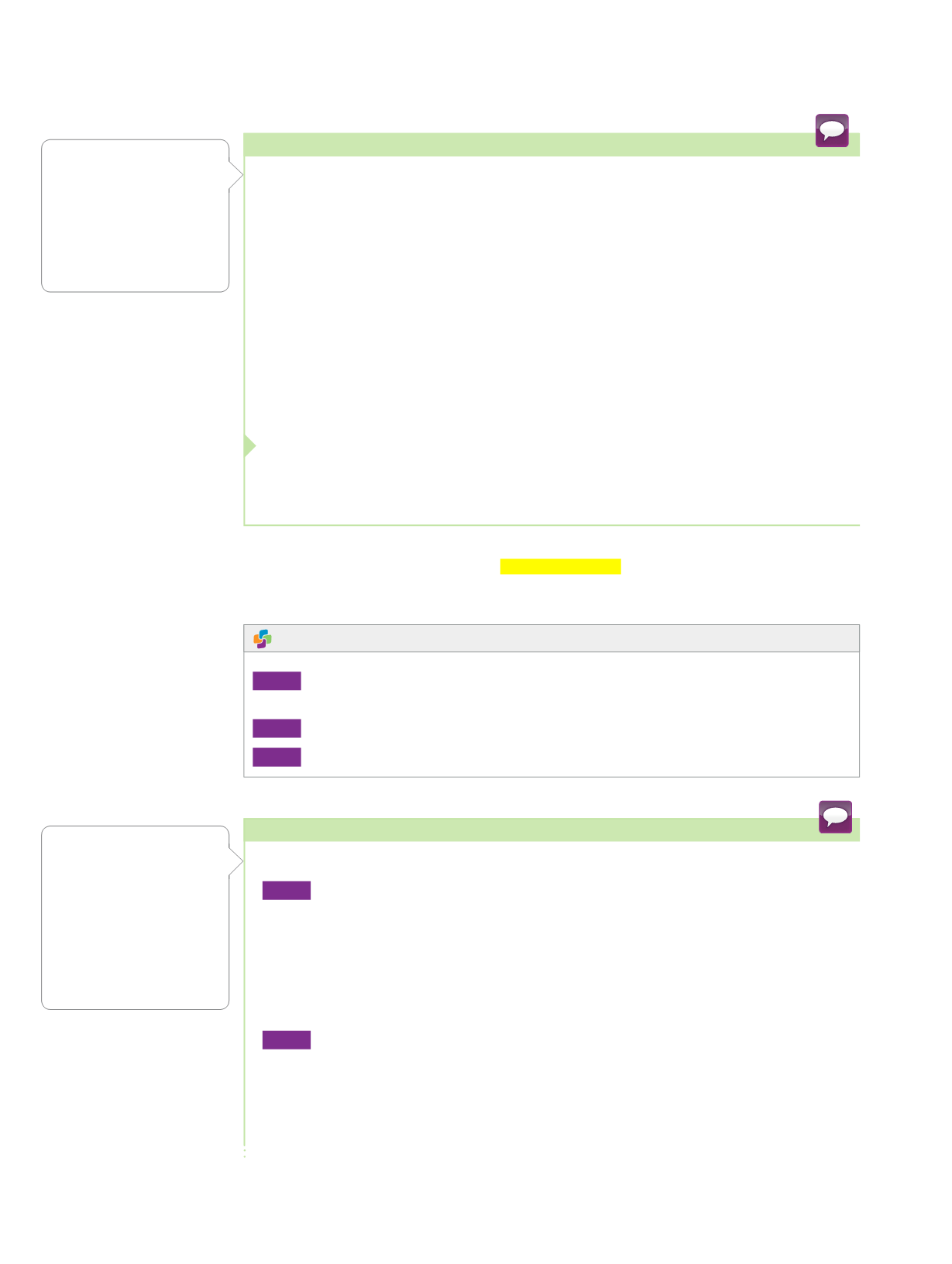
What value of
n
is a solution to 3
(
4
√
2
n
+
6
)
-
6
=
0?
A
-
1
B
1
C
5
D
11
3
(
4
√
2
n
+
6
)
-
6
=
0
Original equation
3
(
4
√
2
n
+
6
)
=
6
Add 6 to each side.
4
√
2
n
+
6
=
2
Divide each side by 3.
(
4
√
2
n
+
6
)
4
=
2
4
Raise each side to the fourth power.
2
n
+
6
=
16
Evaluate each side.
2
n
=
10
Subtract 6 from each side.
n
=
5
The answer is C.
Guided Practice
3.
What value of
x
is a solution of 4(3
x
+
6)
1
_
4
-
12
=
0?
A
x
=
7
B
x
=
25
C
x
=
29
D
x
=
37
Example 3
Solve a Radical Equation
2
Solve Radical Inequalities
A
radical inequality
has a variable in the radicand. To
solve radical inequalities, complete the following steps.
Key Concept
Solving Radical Inequalities
Step 1
If the index of the root is even, identify the values of the variable for which the radicand is
nonnegative.
Step 2
Solve the inequality algebraically.
Step 3
Test values to check your solution.
Example 4
Solve Radical Equations
Solve 3
+
√
5
x
-
10
≤
8.
Step 1
Because the radicand of a square root must be greater than or equal to zero,
first solve 5
x
-
10
≥
0 to identify the values of
x
for which the left side of the
inequality is defined.
5
x
-
10
≥
0
Set the radicand
≥
0.
5
x
≥
10
Add 10 to each side.
x
≥
2
Divide each side by 5.
Step 2
Solve 3
+
√
5
x
-
10
≤
8.
3
+
√
5
x
-
10
≤
8
Original inequality
√
5
x
-
10
≤
5
Isolate the radical.
5
x
-
10
≤
25
Eliminate the radical.
5
x
≤
35
Add 10 to each side.
x
≤
7
Divide each side by 5.
Study Tip
Substitute Values
You
could also solve the multiple-
choice question by
substituting each answer for
n
in the equation to see if
the solution is correct.
Study Tip
Radical Inequalities
Because a principal square
root is never negative,
inequalities that simplify to
the form
√
ax
+
b
≤
c
,
where
c
is a negative
number, have
no solutions.
354
|
Lesson 5-6
|
Solving Radical Equations


















